Where is a function at a high or low point? Calculus can help!
A maximum is a high point and a minimum is a low point:![]()
In a smoothly changing function a maximum or minimum is always where the function flattens out (except for a saddle point).
Where does it flatten out? Where the slope is zero.
Where is the slope zero? The Derivative tells us!
Let’s dive right in with an example:

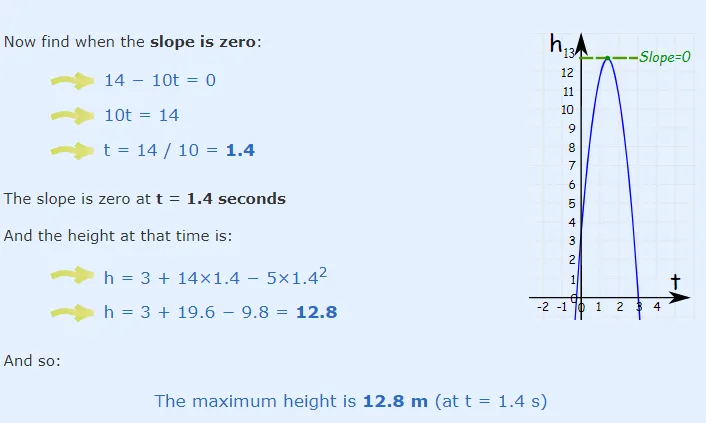
How Do We Know it is a Maximum (or Minimum)?
We saw it on the graph! But otherwise … derivatives come to the rescue again.
Take the derivative of the slope (the second derivative of the original function):
The Derivative of 14 − 10t is −10
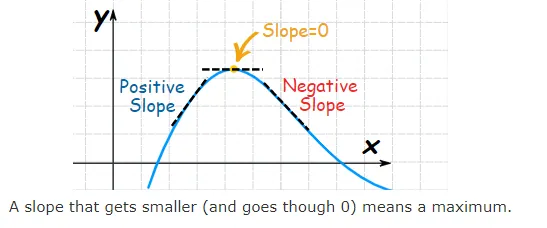
This means the slope is continually getting smaller (−10): travelling from left to right the slope starts out positive (the function rises), goes through zero (the flat point), and then the slope becomes negative (the function falls):
![]()
A slope that gets smaller (and goes though 0) means a maximum.
This is called the Second Derivative Test
On the graph above I showed the slope before and after, but in practice we do the test at the point where the slope is zero:
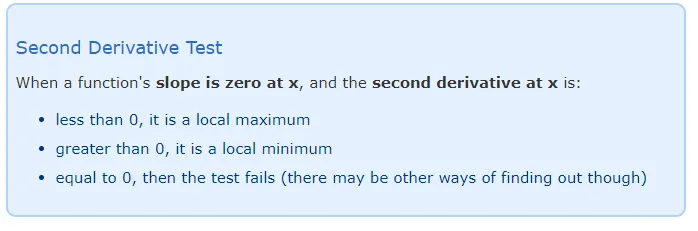
“Second Derivative: less than 0 is a maximum, greater than 0 is a minimum”
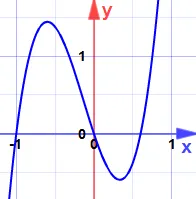
Example: Find the maxima and minima for:
y = 5x3 + 2x2 − 3x
The derivative (slope) is:
![]() y = 15x2 + 4x − 3
y = 15x2 + 4x − 3
Which is quadratic with zeros at:
· x = −3/5
· x = +1/3
Could they be maxima or minima? (Don’t look at the graph yet!)
The second derivative is y” = 30x + 4
At x = −3/5:
y” = 30(−3/5) + 4 = −14
it is less than 0, so −3/5 is a local maximum
At x = +1/3:
y” = 30(+1/3) + 4 = +14
it is greater than 0, so +1/3 is a local minimum
(Now you can look at the graph.)


